-
 Iron bloom
Iron bloom
-
 Wing fairing
Wing fairing
-
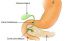 Pancreas
Pancreas
-
 Opportunistic species
Opportunistic species
-
 Multimedia
Multimedia
-
 Cyanosis
Cyanosis
-
 Sedimentation
Sedimentation
-
 Caudiciform
Caudiciform
-
 Capillary texture
Capillary texture
-
 Phase diagram
Phase diagram
-
 Objective
Objective
-
 Ferment
Ferment
-
 Hall effect
Hall effect
-
 Teraflops
Teraflops
-
 Light-year
Light-year
-
 Fertilisation
Fertilisation
-
 Decussation
Decussation
-
 Pigmentary
Pigmentary
-
 Entropy
Entropy
-
 Cloaca
Cloaca
-
 Megacycle
Megacycle
-
 Diverticulum
Diverticulum
-
 To graze
To graze
-
 Random number
Random number
-
 Calciphile
Calciphile
-
 West Nile Virus Disease
West Nile Virus Disease
-
 Abnormality
Abnormality
-
 Internuptial
Internuptial
-
 Oxfam
Oxfam
-
 Cardiopathy
Cardiopathy
Potential energy
When a body is subjected to a force (e.g. a ball falling from a height H above the ground), it can move for a certain distance under the action of this force's work. It can therefore be considered that when the body started moving under the action of the force, it had a reserve of energy, related to the force, which would be used by the work moving the body. This idea is the basis of the concept of potential energy.
If WAB is the work done by the force of interaction (e.g. gravitational or electrostatic) being exerted on the object and moving it from point A to point B, and assuming, as is often the case, that the work does not depend on the path of the object from A to B (this is called a conservative force), then the variation in the potential energy of interaction UAB is equal to the opposite of this work; i.e. -WAB. Then at A the object can be considered to have had a potential energy U(A) and that a part of this energy was used to bring the object from A to B where the potential energy is equal to U(B)
It must be remembered that the force of interaction is not necessarily the force that can be exerted to move an object (itself submitted to a force of interaction). If the applied force is of opposite sign to the force of interaction, this means that in that case WAB= UAB. It is a matter of convention.
The potential energy of a body at a point A is usually defined as the work done by the force applied to this body, and therefore opposed to the force of interaction, required to move the body a finite distance from point A in a given reference frame. This, if the origin of a reference frame is placed at the centre of the Earth, the potential energy U(r) of a body of mass m at a distance r from the centre of the Earth will be equal to:
U(r) = - GmME/r2
where G is the gravitational constant and ME the mass of the Earth.
U(r) = -e2/r2
where e is the electrical charge of an electron.
But in the case of two protons, since the force applied to bring one of the protons from infinity must oppose the electrostatic repulsion, we will have a + sign:
U(r) = +e2/r2
 William John Macquorn Rankine was the Scottish engineer and physicist who introduced the term potential energy. The concept itself goes back to the work of Lagrange. © DR
William John Macquorn Rankine was the Scottish engineer and physicist who introduced the term potential energy. The concept itself goes back to the work of Lagrange. © DR
Latest
Fill out my online form.



